As part of our series on math’s we will focus on answering the question “What is a line of symmetry? And what is its significance and what are its purposes?” as well as giving you all the information you need to help your child understand this small section of the math’s curriculum! Make sure you scroll all the way to the bottom of the blog so that you can find some practice questions for your child to test their skills.

For example, if you cut an apple into two equal halves, then that apple piece is said to be in symmetry with the one next to it. The second example that I would like to give is that if we cut an equilateral triangle into two equal halves, then the two triangles that form after the intersection are right-angled triangles. For line symmetry there are a few more examples, such as square, rectangle, circle, which can also be considered.

Throughout this blog, we will be providing information and resources designed to help parents, teachers, and caregivers to support home learning and find resources to support home learning during the Covid-19 epidemic and beyond.

Introduction of Line of Symmetry

The line of symmetry is an imaginary line that exactly cuts a shape in half.  This means that if you fold the shape along the line, both halves of the shape would match up exactly. In the same way, it would not change the shape if you decided to place a mirror along the line.

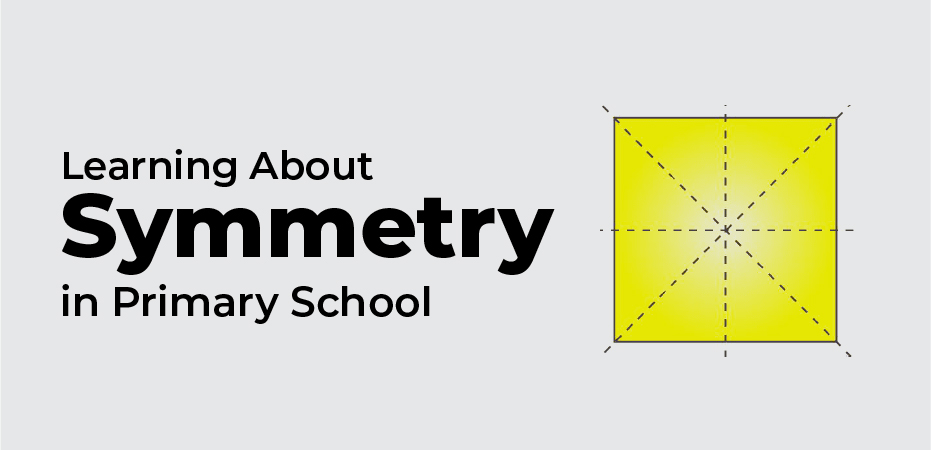
There are four lines of symmetry in a square, as shown in the following illustration.

The symmetry of an equilateral triangle consists of three lines.

Symmetry Lines in Different Symmetrical Shapes

We will be teaching your child about the different shapes and their respective lines of symmetry, but for now, let’s look at some of the most common shapes.

Learning About Symmetry in Primary School

A teacher might ask the child to draw lines of symmetry on the following shapes provided to him or her in Year 2 as an introduction to symmetry. The most common way to make it easier for children to be clear is to give them a cutout shape which they can fold in half so that they can see clearly that both sides of the shape are the same:

Children are asked in Year 4 to identify lines of symmetry in two-dimensional shapes that have been presented in different orientations. The students will need to be aware that shapes can have more than one symmetry line. It may be asked of the students to examine these regular shapes and consider how many lines of symmetry they can find in them. In this instance, it is helpful for them to cut out the shapes and fold them in half, then look at how many folds have been made.

You may also ask the students to classify shapes according to various properties, such as line symmetry. It is possible to give the students a collection of shapes and ask them to place them into a Carroll diagram in the following manner:

The children in the fifth grade begin to reflect shapes in a mirror line. It may be necessary for students to complete the shading in a shape after reflecting it in a mirror line, or for them to draw half of a shape after reflecting it with a mirror line. The shape is usually provided on squared paper in order to allow the students to complete the shape accurately.

Reflected shapes in a mirror line

There will also be an exercise where the children will be given a quarter of a shape and will then be asked to reflect it in two mirror lines, such as:

As an alternative, they can be shown a shape with shaded squares reflected from two mirror lines, and asked to complete the shading of the squares.