Introduction of Vertex Form

A vertex in geometry is a point where two or more curves, lines, or edges meet at a point. Consequently, as a result of this definition, the points of intersection of two lines that form an angle and the corners of polygons and polyhedral are vertices. Each of the four corners of a square, for example, is called a vertex. In other words, it is called by its plural form, vertex. Oftentimes, the word vertex is used as a way to refer to the corners of a polygon.

The angle between two lines at a vertex is called an included angle. When polygons are drawn, the included angle at each vertex is an angle in the interior of the polygon. A vertex is also sometimes used to indicate the ‘top’ or high point of something, such as the vertex of an isosceles triangle, which is the ‘top’ corner opposite the base. However, this is not its precise mathematical meaning.

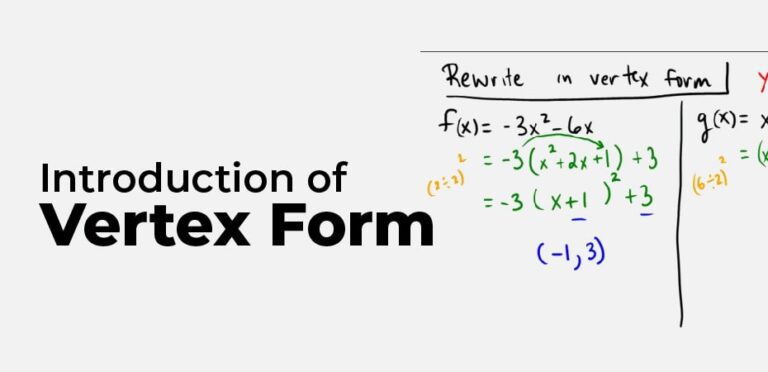
VERTEX FORMAT OF EQUATION:

As a general rule, the vertex form of a parabola’s equation is: y = a(x-h)2+k.

1. This is the vertex (h,k) as you can see in the following image
2. A positive value for a will causes the parabola to open upwards like a regular “U”.
3. In the case of a negative number, the graph opens downwards, like an upside down “U”.
4. In the case of |a|< 1, the graph of the parabola widens. Parabolas have a “U” shape that extends out sideways. With the help of our interactive Parabola Graphed, you can learn more about how ‘a’ works.
5. Ultimately, the graph of the graph becomes narrower if |a|> 1 (That is, the effect of |a|<1 is the opposite).

vertex form calculator

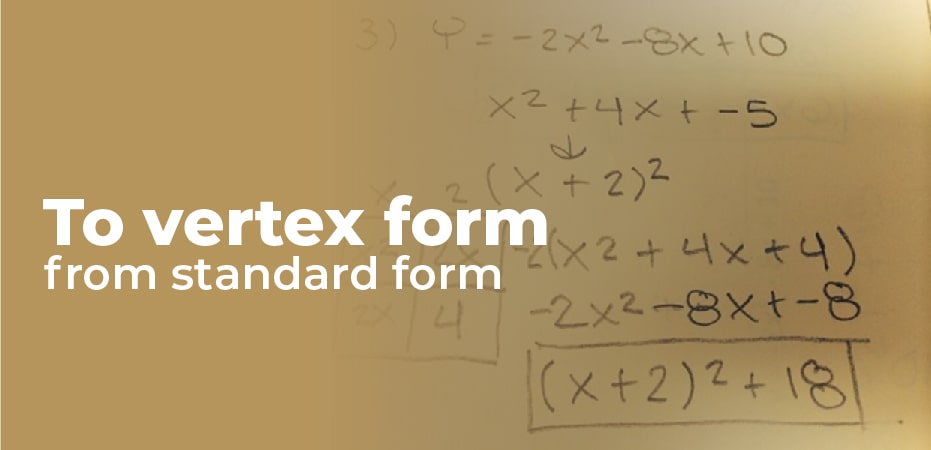
To vertex form from standard form:

Normally, the standard form of a quadratic equation is y = ax^2 + bx + c, where a, b, and c are coefficients, and y and x are variables. If you need to graph a quadratic function, or parabola, the process is streamlined when the equation is in vertex form because you can quickly find the solution with a, b, and c. However, if you need to graph a quadratic function, or parabola, the process is streamlined when the equation is in vertex form. Y is the vertex form of a quadratic equation when it is m(x-h)^2 + k, where m represents the slope of the line, and h and k represent any point on the line.

Coefficient of Factorization:

The coefficient a is taken as the product of the first two terms of the standard form equation. Place the coefficient outside the parentheses. The process of factoring quadratic equations in standard form entails finding two numbers that are added together to equal b and multiplied together to equal ac. For instance, if you are converting 2x^2 – 28x + 10 to vertex form, you first need to write 2(x^2 – 14x) + 10.

Coefficient of division:

The next step is to divide the coefficient of the x term within parentheses by two. Once this has been done, it will then be squared using the square root property. It is possible to find a quadratic equation solution using the square root property method by taking the square roots of both sides. According to the example, the coefficient of the x inside the parentheses is -14.

Equation of Balance

For balance, multiply the number inside the parentheses by the factor outside the parentheses and subtract it from the whole quadratic equation. For example, 2(x^2 – 14x) + 10 becomes 2(x^2 – 14x + 49) + 10 – 98, since 49*2 = 98. You can simplify the equation by combining the terms at the end. For example, 2(x^2 – 14x + 49) – 88, since 10 – 98 = -88.