The radius formula can simply be derived by halving the diameter of the circle. If we make a line segment that connects a point on the circumference of a circle to the exact center of the circle, then the line segment that we make is called the radius of the circle. It is the distance between the center of a circle and the circumference of the circle that is called its radius. In all cases, the diameter is twice the radius. This means that the diameter is divided by two in order to obtain the formula.
Intro of Radius
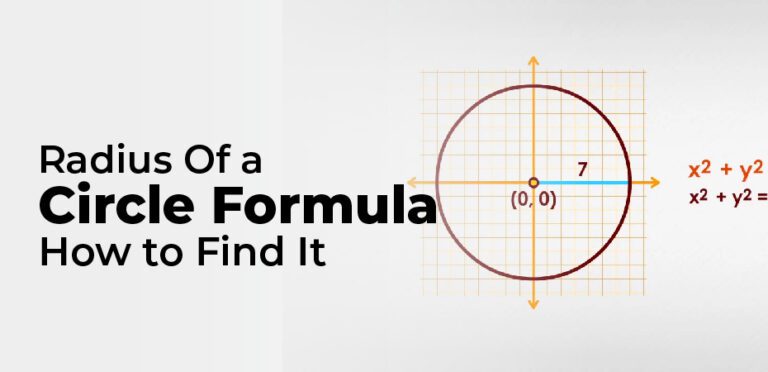
In geometry, a radius is defined as the line segment connecting the center of a circle or sphere with its circumference or boundary. This is generally abbreviated as ‘r’, which is an important part of circles and spheres. Whenever we talk about more than one radius at a time, we use the plural “radii”. In circles or spheres, the diameter is the longest line segment connecting any two points lying on the opposite side of the center, and the radius is half the diameter. This can be expressed as d/2, where d is the diameter of the circle or sphere. The image below shows the relationship between diameter and radius for a circle.
With the given information, let’s learn how to calculate the radius’s length using the formulas.
Formulas for Radius
Radius Formula in terms of diameter, circumference, and area is as follows:
Radius Formulas
Radius in Terms of Diameter d ⁄ 2
Radius in Terms of Circumference C ⁄ 2π
Radius in Terms of Area √(A ⁄ π)
Where,
- r is the radius of the circle
- d is the diameter of the circle
- C is the circumference of the circle
- A is the area of the circle
The Radius of a Circle – How to Find
There are three basic formulas for finding a circle’s radius, i.e., when the diameter, area, or circumference are known. Here is the formula for finding the radius of a circle
- If you know the diameter, the formula is Radius = Diameter/2.
- When the circumference of an object is known, the radius can be calculated by using Radius = Circumference/2π.
- For an area of a circle that is known, the radius can be calculated using the formula Radius =⎷(Area of the circle/π).
As an example, if we specify 24 units for the diameter, then the radius will be 24/2 = 12 units. A circle’s radius can be calculated as 44/2π if the circumference of the circle is 44 units. This implies, (44×7)/(2×22) = 7 units. And, if the area of a circle is given as 616 square units, then the radius is ⎷(616×7)/22 = ⎷28×7 = ⎷196 = 14 units.
Radius of a Sphere
An object that is 3D solid is a sphere. It is the segment from any point on the boundary of the sphere to its center. It is a determining factor when drawing a sphere, since its size is determined by its radius. A sphere can have an infinite number of radii and they will all have the same length, just as circles do. We need to know the radius of a sphere in order to calculate its volume and surface area. Our formulas for volume and surface area make it easy to calculate the radius of the sphere.
Radius of the sphere from volume = 3⎷(3V)/4π units, where V represents the volume and the value of π is approximately 3.14.
Radius of a sphere in surface area units = ⎷(A/4π) units, where A represents the surface area.
Use our free online sphere radius calculator to calculate the radius given a volume, surface area, or diameter of a sphere.